2.7.2 Spectral Experiment II

1. Hydrogen lamp 2. Spectrum of the hydrogen lamp
Hydrogen lamp Spectrum of the hydrogen lamp
Photo 1: The photo shows a hydrogen lamp. Hydrogen molecules in the lamp are dissociated into excited hydrogen atoms using an electron ray. The molecules then give up their energy of excitation in the form of electromagnetic radiation. Some of this electromagnetic radiation lies in the area of visible light.
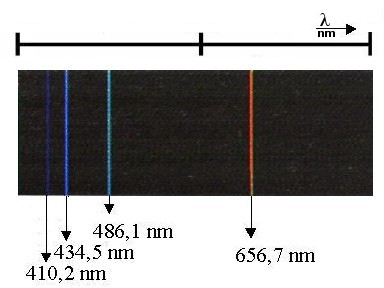
Photo 2: The hydrogen lamp does not produce a continuous spectrum, in contrast to the Wolfram lamp depicted in Experiment 2.7.1. Rather, it produces discreet lines. Four of these spectral lines are in the range of visible light. The rest lie in the ultraviolet and infrared regions. Out of the four present in the visible light area, red appears the brightest, which is why the hydrogen lamp appears red to the naked eye.
The spectrum shown here is part of the so-called Balmer series, whose regularity was discovered by the Swiss teacher Johann Jakob Balmer at the end of the 19th century from experiments. The general relation according to which all lines of the hydrogen spectrum can be construction, was discovered by the Swedish spectroscoper Johannes Rydberg. The formula he discovered can be used to calculate the frequency of all the emission lines of hydrogen:

, , n=whole number, n2 > n1
(For the Balmer series, n2=2)
The Balmer series and the Rydberg formula were an important basis for the construction of the Bohr model of the atom.
 
Exercise 2.7.1.2:
Solution		 Bohr discovered an expression for the Rydberg constant or Rydberg frequency using constants of nature:

RH=(me×e4 / 8x3 × e02)

where
e0 = dielectric constant (permittivity) in a vacuum, 8.85 × 10-12 C2/(J m), 
e= charge of an electron, 1.602×10-19 C
me= mass of an electron, 9.11×10-31 kg

What units does the factor x have? What constant of nature is it?
 
Exercise 2.7.1.3:
Solution		 What is the wavelength of the photon emitted from a transition between states n1=2 to n2=4?

back